6.5.3 Times series and their uncertainties
Study of the monthly dispersion
The SMM/ACRIM I daily mean values with their standard
deviations (thereafter STD) are plotted in Figure
6.11a for the time
interval of 1980 to 1989. The vertical error bars in Figure
6.11a
indicate the standard deviations of the mean of the irradiance
samples obtained within a day. To study the relation between the
data and their uncertainty, the STD of the SMM/ACRIM I data versus
their daily means are presented in Figure
6.11b. As can be seen from
Figure 6.11a,
total solar irradiance continuously decreased from the
maximum of solar cycle 21 (1980) towards solar minimum and started
to rise in early 1987 with the ascending phase of solar cycle 22
(Willson and Hudson, 1988). The increased measuring uncertainty
during the spin operational mode of the SMM satellite is clearly
seen in Figure 6.11a.
In order to study the variability of STD in more
detail, the normal operational and spin-mode data have been
separated and their STD has been plotted against the daily mean
values in Figures
6.12a,b,
respectively. While no particular structure
can be seen in the case of the spin-mode data
(Figure 6.12b), the
ACRIM I data during the normal operational mode
(Figure 6.12a) form a
certain pattern on the scatter plot diagram of their standard
deviation. Most of the irradiance values (95%) are concentrated
below 0.05 
STD,
whereas the outliers from that main cluster
(STD>0.05) tend to be distributed around two vertical lines.
To further investigate the physical nature of the pattern
observed in the normal mode of ACRIM I data, the quiet- and
active-Sun irradiance values and their standard deviations have
been separated. The quiet-Sun irradiance values have been choosen
for the time interval of July 1, 1984 to September 30, 1986, when
total solar irradiance showed only a small variability around its
mean value. There was an obvious decline in solar activity in July
1984 and after this time only small sunspots occurred on the Sun.
Although the magnetic solar minimum occurred in September
1986, in October large active regions, already belonging to solar
cycle 22, were formed on the Sun and caused significant variability
in both UV and total solar irradiances (Pap, Marquette and
Donnelly, 1991). Irradiance values between February and December
1980 and after October 1, 1986 have been identified as active-Sun
values in this phase of our study.
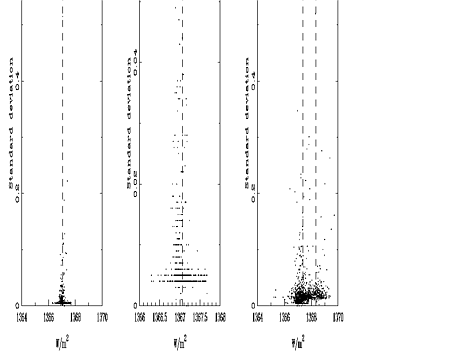
The scatter plot diagram of the quiet-Sun irradiance values
and their standard deviations are presented in Figure
6.13a. The
vertical line represents the mean value of the quiet-Sun irradiance
(1367.07 ).
Figure 6.13b consists of a
particular part of the
quiet-Sun data with STD smaller than 0.06
. Most of these
data points (
74%)
are concentrated between STD 0.004 and
0.005 and these
numbers essentially represent the intrinsic
precision of the ACRIM I data (corresponding to 0.0004%), in a
good agreement with the published value for the average uncertainty
of the ACRIM I data (better than
0.002%; Willson, 1984). The
structures seen in Figures
6.13a,b represent a Gaussian distribution
of the data around their mean value. Since during solar minimum
only small variability is observed in total irradiance, data points
above 0.01 STD
represent the random noise in the data. The
active-Sun values and the corresponding standard deviations are
plotted in Figure 6.13c and these
data show a very similar pattern to
that seen in Figure6.12a
(data points plotted for the whole normal
operational time interval). Namely, data points with STD larger
than 0.05 are again distributed around two vertical lines. The
first vertical line is drawn at the mean irradiance value (M =
1367.36 )
for the rising portion of solar cycle 22 between
October 1, 1986 and June 2, 1989, the end of the ACRIM I data set.
The second vertical line represents the mean irradiance value for
the maximum of solar cycle 21 in 1980 (M = 1368.35
). These
two distributions represent most closely Gaussian distributions and
these data (STD>0.05) can again be regarded as random
fluctuations in the data.
Another interesting feature of Figures
6.12 and
6.13 is that the standard
deviation less than 0.05
increases with the increasing
daily mean values of total irradiance from minimum to maximum
activity conditions. The higher irradiance values represent maximum
solar activity conditions (Willson and Hudson, 1988; Foukal and
Lean, 1988), when significant changes may occur within a day due to
the effect of flares as well as the formation and evolution of
active regions, especially sunspots. In contrast, during minimum
activity conditions, when only a few and small active regions
occur on the Sun, variations in total solar irradiance within a day
are small, and this may explain the lower standard deviation of the
ACRIM I total solar irradiance values during solar minimum.
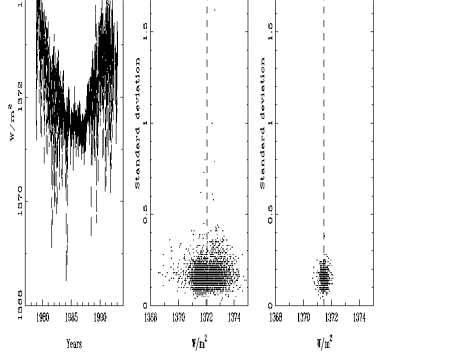
The daily Nimbus-7/ERB irradiance values with their standard
deviations are presented in Figure
6.14a. The ERB STD values versus
the daily means are shown in Figure
6.14b. As can be seen from
Figure 6.14, the uncertainty
of the Nimbus data is much higher than
that of the ACRIM I data (except for the spin-mode ACRIM I data)
due to the lack of a solar pointing platform and the limited number
of observations (Hickey et al., 1988). While in the case of
the normal operation of the ACRIM experiment more than 800
observations were taken within a day (Willson, 1984), the ERB
experiment had a 3-day-on and 1-day-off operational mode through
most of its operation and the number of observations was limited to
less than a dozen per day (Hickey et al., 1988). The
Nimbus-7/ERB irradiance values do not show the pattern seen in the
case of the normal mode of ACRIM data, instead they form a large
cloud and the data are evenly distributed between STD = 0.05 and
0.5 similar to the spin-mode ACRIM I data.
Figure 6.14c
represents the distribution of the quiet-Sun ERB values (for the
same time interval as choosen for the ACRIM I data). Although
higher irradiance values represent maximum activity
conditions similar to the ACRIM I observations, the Gaussian
distribution, that was clearly seen in the case of the normal mode
of ACRIM I data, is not recognized in the ERB data because of their
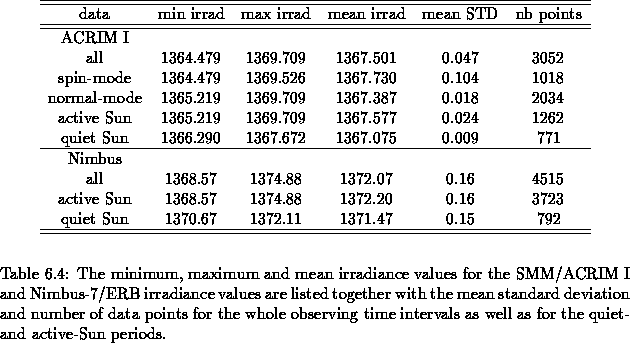
larger uncertainty. The mean STD values for both the ERB and ACRIM
I total irradiance during the quiet- and active-Sun conditions are
listed in Table 6.4.
Table 6.4 also contains the maximum, minimum and
mean quiet- and active-Sun irradiance values as well as the number
of the corresponding data points.
6.5.3 Times series and their uncertainties
Study of the monthly dispersion
vig@
Fri Nov 1 16:09:33 MET 1996